“Ichnos” – CNC Milled Generative Ornament

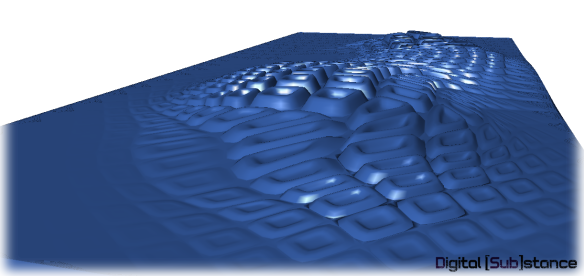
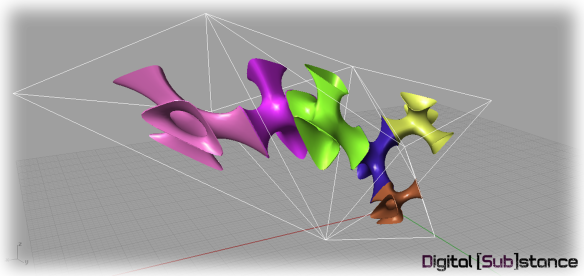
“Ichnos v.01” is the first CNC milled derivative of the “Ichnos” series, a project I have been working on in collaboration with Wade Martin of AxisCutParts in Auburn Washington. Ichnology  is the branch of geology that deals with traces of organismal behavior, such as burrows and footprints. “Ίχνος” is the trace in ancient and modern Greek. The design of Ichnos is based on the traces of  grey-scale image samples, those traces are deformed , tessellated, distorted and finally smoothened into embossed meshes. The algorithm is developed in Grasshopper3D, contains some VB.Net scripting and part of it can be accessed here.

The produced geometry is exported from rhino, then imported into the CNC software where using surface machining processes is roughed out with .125 inch call nose, while  the finish pass is made with a .0625 inches by a step-over of .003 inches. The overall process took over 4 hours to be completed. The material used in the sample is a solid 12 mm thick white corian surface of 5×10 inches.

TetraAggregationV2.0 | Aggregating Systems using Hoopsnake for Grasshopper3d

[vimeo http://vimeo.com/38565641 w=620&h=341]

This is a follow-up to my last post on tetrahedral morphing using Grasshopper3d and some VB.net. I implemented this process into a recursive methodology using the Hoopsnake Component for GH developed by Yiannis Chatzikonstantinou, in order to put together a dynamically growing system. The process utilizes a method similar to DLA (Diffuse Limited Aggregation) with the significant difference of being sustained in only one face of the tetrahedron each time. This way the  aggregated geometry remains non self-intersecting. The results of this process are quite interesting mostly because of the emergent characteristics of the recursion .

You can grab the code from here.

Enjoy!

TetraAggregation | Tetrahedral Morphing in Grasshopper

Box Morphing is a common technique used in GH  to create panelled surfaces or evenly packed geometries. Tetrahedral morphing however is not available through a single component. Going through  a very interesting discussion in the GH forum, I decided to address a simple aggregation system through Tetrahedral Morphing. The definition implements a 4×4 transformation matrix [Math Power!!!!] to morph an initial geometry to a provided set of tetrahedra. Moreover, some VB.Net scripting is essential here for the development of the matrix (originally written by Jacek Jaskólski \ re-written narrowed down by myself). Tetrahedral morphing can be extremelly interesting in the case of  Barycentric coordinates, follow the GH discussion if you want to get into more detail on that .

In TetraAggregation the initial morphed geometry is a 4-axial relaxed surface dependable on 4 points(thus a tetrahedron). This is geometry is consequentiality morphed into a  group face-attached tetrahedra to create the gap-less aggregation result.

you can download the def here.